What We Cannot Know

A BUTTERFLY CALLED MERCURY

Poincaré wasn’t able to answer the King of Sweden’s question about the solar system: whether it would remain in a stable equilibrium or might fly apart in a catastrophic exhibition of chaotic motion. His discovery that some dynamical systems can be sensitive to small changes in data opened up the possibility that we may never know the precise fate of the solar system much in advance of any potentially devastating scenario unfolding.

It is possible that, like population dynamics with a low reproductive rate, the solar system is in a safe predictable region of activity. Unfortunately, the evidence suggests that we can’t console ourselves with this comforting mathematical hope. Recent computer modelling has provided new insights which reveal that the solar system is indeed within a region dominated by the mathematics of chaos.

I can measure how big an effect a small change will have on the outcome using something called the Lyapunov exponent. For example, in the case of billiards played on differently shaped tables, I can give a measure of how catastrophic a small change will be on the evolution of a ball’s trajectory. If the Lyapunov exponent of a system is positive, it means that if I make a small change in the initial conditions then the distance between the paths diverges exponentially. This can be used as a definition of chaos.

With this measure several groups of scientists have confirmed that our solar system is indeed chaotic. They have calculated that the distance between two initially close orbital solutions increases by a factor of ten every 10 million years. This is certainly on a different timescale to our inability to predict the weather. Nevertheless, it means that I can have no definite knowledge of what will happen to the solar system over the next 5 billion years.

If you’re wondering in despair whether we can know anything about the future, then take heart in the fact that mathematics isn’t completely hopeless at making predictions. There is an event that the equations guarantee will occur if we make it to 5 billion years from now, but it’s not good news: the mathematics implies that at this point the Sun will run out of fuel and evolve into a red giant engulfing planet Earth and the other planets in our solar system in the process. But until this solar blowout engulfs the solar system, I am faced with trying to solve chaotic equations if I want to know which planets will still be around to see that red giant.

This means that, like the predictions of the weather, if I want to know what is going to happen, I am reduced to running simulations in which I vary the precise locations and speeds of the planets. The forecast is in some cases rather frightening. In 2009 French astronomers Jacques Laskar and Mickael Gastineau ran several thousand models of the future evolution of our solar system. And their experiments have identified a potential butterfly: Mercury.

The simulations start by feeding in the records we have of the positions and velocities of the planets to date. But it is difficult to know these with 100% accuracy. So each time they run the simulation they make small changes to the data. Because of the effects of chaos theory, just a small change could result in a large deviation in the outcomes.

For example, astronomers know the dimensions of the ellipse of Mercury’s orbit to an accuracy of several metres. Laskar and Gastineau ran 2501 simulations where they varied these dimensions over a range of less than a centimetre. Even this small perturbation resulted in startlingly different outcomes for our solar system.

You might expect that if the solar system was going to be ripped apart it would have to be one of the big planets like Jupiter or Saturn that would be the culprit. But the orbits of the gas giants are extremely stable. It’s the rocky terrestrial planets that are the troublemakers. In 1% of simulations that they ran, it was tiny Mercury that posed the biggest risk. The models show that Mercury’s orbit could start to extend due to a certain resonance with Jupiter, with the possibility that Mercury could collide with its closest neighbour, Venus. In one simulation, a close miss was enough to throw Venus out of kilter, with the result that Venus collides with Earth. Even close encounters with the other planets would be enough to cause such tidal disruption that the effect would be disastrous for life on our planet.

This isn’t simply a case of abstract mathematical speculation. Evidence of such collisions has been observed in the planets orbiting the binary star Upsilon Andromedae. Their current strange orbits can be explained only by the ejection of an unlucky planet sometime in the star’s past. But before we head for the hills, the simulations reveal that it will take several billion years before Mercury might start to misbehave.

INFINITE COMPLEXITY

What of my chances to predict the throw of the dice that sits next to me? Laplace would have said that, provided I can know the dimensions of the dice, the distribution of the atoms, the speed at which it is launched, its relationship to its surrounding environment, theoretically the calculation of its resting point is possible.

The discoveries of Poincaré and those who followed have revealed that just a few decimal places could be the difference between the dice landing on a 6 or a 2. The dice is designed to have only six different outcomes, yet the input data ranges over a potentially continuous spectrum of values. So there are clearly going to be points where a very small change will flip the dice from landing on a 6 to a 2. But what is the nature of those transitions?

Computer models can produce very good visual representations that give me a handle on the sensitivity of various systems to the starting conditions. Next to my Vegas dice I’ve got a classic desktop toy that I can play with for hours. It consists of a metal pendulum that is attracted to three magnets, coloured white, black and grey. Analysis of the dynamics of this toy has led to a picture that captures the ultimate outcome of the pendulum as it starts over each point in the square base of the toy. Colour a point white if starting the pendulum at this point results in it ending at the white magnet. Similarly, colour the point grey or black if the ultimate destination is grey or black. This is the picture you get:

As in the case of population dynamics, there are regions which are entirely predictable. Start close to a magnet and the pendulum will just be attracted to that magnet. But towards the edges of the picture I find myself in far less predictable terrain. Indeed, the picture is now an example of a fractal.

There are regions where there isn’t a simple transition from black to white. If I keep zooming in, the picture never becomes just a region filled with one colour. There is complexity at all scales.

A one-dimensional example of such a picture can be cooked up as follows. Draw a line of unit length and begin by colouring one half black and the other white. Then take half the line from the point 0.25 to 0.75 and flip it over. Now take the half in between that and flip it over again. If we keep doing this to infinity then the predicted behaviour around the point at 0.5 is extremely sensitive to small changes. There is no region containing the point 0.5 which has a single colour.

There is a more elaborate version of this picture. Start again with a line of unit length. Now rub out the middle third of the line. You are left with two black lines with a white space in between. Now rub out the middle third of each of the two black lines. Now we have a black line of length 1⁄9, a white line of length 1⁄9, a black line of length 1⁄9, then the white line of length 1⁄3 that was rubbed out on the first round, and then a repeat of black–white–black.

You may have guessed what I am going to do next. Each time rub out the middle third of all the black lines that you see. Do this to infinity. The resulting picture is called the Cantor set, after the German mathematician Georg Cantor, whom we will encounter in the last Edge, when I explore what we can know about infinity. Suppose this Cantor set was actually controlling the outcome of the pendulum in my desktop toy. If I move the pendulum along this line, I find that this picture predicts some very complicated behaviour in some regions.

A rather strange calculation shows that the total length of the line that has been rubbed out is 1. But there are still black points left inside: 1⁄4 is a point that is never rubbed out, as is 3⁄10. These black points, however, are not isolated. Take any region round a black point and you will always have infinitely many black and white points inside the region.

What do the dynamics of my dice look like? Are they fractal and hence beyond my knowledge? My initial guess was that the dice would be chaotic. However, recent research has turned up a surprise.

KNOWING MY DICE

A Polish research team has recently analysed the throw of a dice mathematically, and by combining this with the use of high-speed cameras they have revealed that my dice may not be as chaotic and unpredictable as I first feared. The research group consists of father-and-son team Tomasz and Marcin Kapitaniak together with Jaroslaw Strzalko and Juliusz Grabski, and they are based in Lódź. In their paper in the journal Chaos, published in 2012, the team draw similar pictures to those for the magnetic pendulum, but the starting positions are more involved than just two coordinates because they have to give a description of the angle at which the cube is launched and also the speed. The dice will be predictable if for most points in this picture when I alter the starting conditions a little the dice ends up falling on the same side. I can think of the picture being coloured by six colours corresponding to the six sides of the dice. The picture is fractal if however much I zoom in on the shape I still see regions containing at least two colours. The dice is predictable if I don’t see this fractal quality.

The model the Polish team considered assumes the dice is perfectly balanced like the dice I brought back from Vegas. Air resistance, it turns out, can be ignored as it has very little influence on the dice as it tumbles through the air. When the dice hits the table a certain proportion of the dice’s energy is dissipated, so that after sufficiently many bounces the dice has lost all kinetic energy and comes to rest.

Friction on the table is also key, as the dice is likely to slide in the first few bounces but won’t slide in later bounces. However, the model explored by the Polish team assumed a frictionless surface as the dynamics get too complicated to handle when friction is present. So imagine throwing the dice onto an ice rink.

I’d already written down equations based on Newton’s laws of motion for the dynamics of the dice as it flies through the air. In the hands of the Polish team they turn out not to be too complicated. It is the equations for the change in dynamics after the impact with the table that are pretty frightening, taking up ten lines of the paper they wrote.

They discovered that if the amount of energy dissipated on impact with the table is quite high, the picture of the outcome of the dice does not have a fractal quality. This means that if one can settle the initial conditions with appropriate accuracy, the outcome of the throw of my dice is predictable and repeatable. This predictability implies that, more often than not, the dice will land on the face that was lowest when the dice is launched. A dice that is fair when static may actually be biased when one adds in its dynamics.

But as the table becomes more rigid, resulting in less energy being dissipated and hence the dice bouncing more, I start to see a fractal quality emerging.

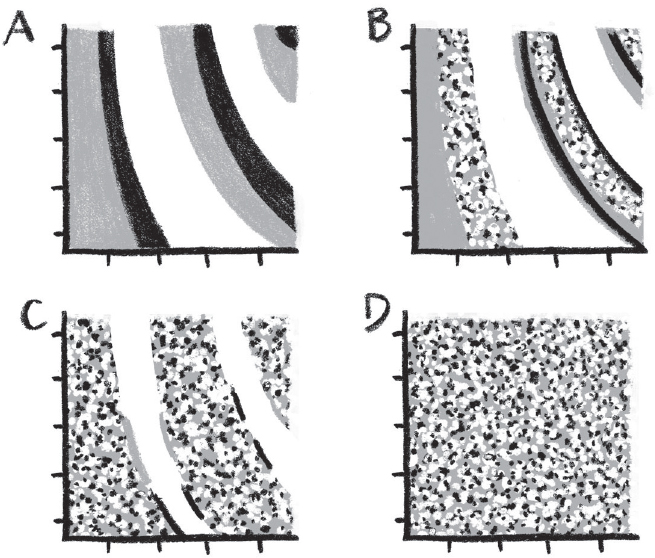
Moving from (a) to (d), the table dissipates less energy, resulting in a more fractal quality for the outcome of the dice.

This picture looks at varying two parameters: the height from which the dice is launched and variations in the angular velocity around one of the axes. The less energy that is dissipated on impact with the table, the more chaotic its resulting behaviour and the more it seems that the outcome of my dice recedes back into the hands of the gods.

DOES GOD PLAY DICE?

What of the challenge to define God as the things we cannot know? Chaos theory asserts that I cannot know the future of certain systems of equations because they are too sensitive to small inaccuracies. In the past gods weren’t supernatural intelligences living outside the system but were the rivers, the wind, the fire, the lava – things that could not be predicted or controlled. Things where chaos lay. Twentieth-century mathematics has revealed that these ancient gods are still with us. There are natural phenomena that will never be tamed and known. Chaos theory implies that our futures are often beyond knowledge because of their dependence on the fine-tuning of how things are set up in the present. Because we can never have complete knowledge of the present, chaos theory denies us access to the future. At least until that future becomes the present.

That’s not to say that all futures are unknowable. Very often we are in regions which aren’t chaotic and small fluctuations have little effect on outcomes. This is why mathematics has been so powerful in helping us to predict and plan. Here we have knowledge of the future. But at other times we cannot have such control, and yet this unknown future will certainly impact on our lives at some point.

Some religious commentators who know their science and who try to articulate a scientific explanation for how a supernatural intelligence could act in the world have intriguingly tried to use the gap that chaos provides as a space for this intelligence to affect the future.

One of these religious scientists is the quantum physicist John Polkinghorne. Based at the University of Cambridge, Polkinghorne is a rare mind who combines both the rigours of a scientific education with years of training to be a Christian priest. I will be meeting Polkinghorne in person in the Third Edge when I explore the unknowability inherent in his own scientific field of quantum physics. But he has also been interested in the gap in knowledge that the mathematics of chaos theory provides as an opportunity for his God to influence the future course of humanity.

Polkinghorne has proposed that it is via the indeterminacies implicit in chaos theory that a supernatural intelligence can still act without violating the laws of physics. Chaos theory says that we can never know the set-up precisely enough to be able to run deterministic equations, and hence there is room in Polkinghorne’s view for divine intervention, to tweak things to remain consistent with our partial knowledge but still influence outcomes.

Polkinghorne is careful to stress that to use infinitesimal data to effect change requires a complete holistic top-down intervention. This is not a God in the detail but by necessity an all-knowing God. Given that chaos theory means that even the location of an electron on the other side of the universe could influence the whole system, we need to have complete, holistic knowledge of the whole system – the whole universe – to be able to steer things. We cannot successfully isolate a part of the universe and hope to make predictions based on that part. So it would require knowledge of the whole to act via this chink in that which is unknown to us.

Chaos theory is deterministic, so this isn’t an attempt to use the randomness of something like quantum physics as a way to have influence. Polkinghorne’s take on how to square the circle of determinism and influence the system is to use the gap between epistemology and ontology, between what we know and what is true. Since we cannot know a complete description of the state of the universe at this moment in time, this implies that from our perspective there is no determinacy. There are many different scenarios that coincide with our impartial description of what we currently know about how the universe is set up. Polkinghorne’s contention is that at any point in time this gives a God the chance to intervene and shift the system between any of these scenarios without us being aware of the shift. But, as we have seen, chaos theory means that these small shifts can still have hugely different outcomes. Polkinghorne is careful to assert that you allow shifts between systems where there is change only in information, not energy. The rule here is not to violate any rules of physics. As Polkinghorne says: ‘The succession of the seasons and the alternations of day and night will not be set aside.’

Even if you think this is rather fanciful (which I certainly do), a similar principle is probably key to our own feeling of agency in the world. The question of free will is related ultimately to questions of a reductionist philosophy. Free will describes the inability to make any meaningful reduction in most cases to an atomistic view of the world. So it makes sense to create a narrative in which we have free will because that is what it looks like on the level of human involvement in the universe. If things were so obviously deterministic, with little variation to small undetectable changes, we wouldn’t think that we had free will.

It is striking that Newton, the person who led us to believe in a clockwork deterministic universe, also felt that there was room in the equations for God’s intervention. He wrote of his belief that God would sometimes have to reset the universe when things looked like they were going off course. He got into a big fight with his German mathematical rival Gottfried Leibniz, who couldn’t see why God wouldn’t have set it up perfectly from the outset:

Sir Isaac Newton and his followers have also a very odd opinion concerning the work of God. According to their doctrine, God Almighty wants to wind up his watch from time to time: otherwise it would cease to move. He had not, it seems, sufficient foresight to make it a perpetual motion.

ON THE EDGE OF CHAOS

Newton and his mathematics gave me a feeling that I could know the future, that I could shortcut the wait for it to become the present. The number of times I have heard Laplace’s quote about ultimately being able to know everything thanks to the equations of motion is testament to a general feeling among scientists that the universe is theoretically knowable.

The mathematics of the twentieth century revealed that theory doesn’t necessarily translate into practice. Even if Laplace is correct in his statement that complete knowledge of the current state of the universe together with the equations of mathematics should lead to complete knowledge of the future, I will never have access to that complete knowledge. The shocking revelation of twentieth-century chaos theory is that even an approximation to that knowledge won’t help. The divergent paths of the chaotic billiard table mean that since we can never know which path we are on, our future is not predictable.

Chaos theory implies that there are things we can never know. The mathematics in which I had placed so much faith to give me complete knowledge has revealed the opposite. But it is not entirely hopeless. Many times the equations are not sensitive to small changes and hence give me access to predictions about the future. After all, this is how we landed a spaceship on a passing comet. Not only that: as Bob May’s work illustrates, the mathematics can even help me to know when I can’t know.

But a discovery at the end of the twentieth century even questions Laplace’s basic tenet of the theoretical predictability of the future. In the early 1990s a PhD student by the name of Zhihong Xia proved that there is a way to configure five planets such that when you let them go, the combined gravitational pull causes one of the planets to fly off and reach an infinite speed in a finite amount of time. No planets collide, but still the equations have built into them this catastrophic outcome for the residents of the unlucky planet. The equations are unable to make any prediction of what happens beyond this point in time.

Xia’s discovery is a fundamental challenge to Laplace’s view that Newton’s equations imply that we can know the future if we have complete knowledge of the present, because there is no prediction even within Newton’s equations for what happens next for that unlucky planet once it hits infinite speed. The theory hits a singularity at this point, beyond which prediction makes no sense. As we shall see in later Edges, considerations of relativity will limit the physical realization of this singularity since the unlucky planet will eventually hit the cosmic speed limit of the speed of light, at which point Newton’s theory is revealed to be an approximation of reality. But it nevertheless reveals that equations aren’t enough to know the future.

It is striking to listen to Laplace on his deathbed. As he sees his own singularity heading towards him with only a finite amount of time to go, he too admits: ‘What we know is little, and what we are ignorant of is immense.’ The twentieth century revealed that, even if we know a lot, our ignorance will remain immense.

But it turns out that it is isn’t just the outward behaviour of planets and dice that is unknowable. Probing deep inside my casino dice reveals another challenge to Laplace’s belief in a clockwork deterministic universe. When scientists started to look inside the dice to understand what it is made of, they discovered that knowing the position and the movement of the particles that make up the dice may not even be theoretically possible. As I shall discover in the next two Edges, there might indeed be a game of dice at work that controls the behaviour of the very particles that make up my red Las Vegas cube.